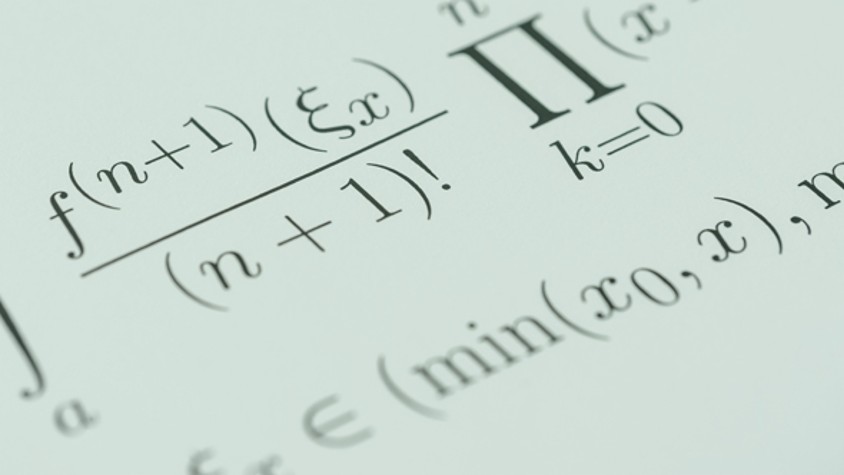Research Group

Welcome!

As Numerical Analysis and Scientific Computing group we work on numerical methods, mainly for partial differential equations. We are happy about your interest, feel free to contact us! Spokesperson: Tabea Tscherpel (WiSe 25/26)

On the research level we develop efficient and robust numerical schemes, for example by means of structure preservation. For such schemes we deal with convergence analysis and both a priori and a posteriori error estimates. In situations of uncertain data we investigate techniques for uncertainty quantification and data assimilation that can be incorporated in numerical schemes. Efficiency can be enhanced by model order reduction as well as adaptivity, both on the level of the model and on the level of the mesh.

Most partial differential equations we work on are coming from compressible fluid dynamics. Applications include for example gas networks, compressible Euler equations and non-Newtonian fluids. Also partial differential equations from other fields such as electrodynamics, geothermics, medicine and biology are considered.

On the other hand for teaching we offer a wide range of courses for students on various aspects of numerical approximation. Notably, we aim for a solid basic education that will be practical and useful for anyone. Building on that, we enjoy offering specialisation in areas with close connections to our research.

Overview